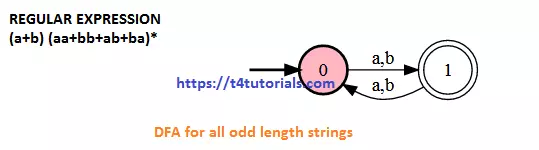
Write the Regular expression for the language of all odd-length strings defined over ∑ = {a, b}?
Specifying odd length with regular expression
Valid Strings:
These all strings are the part of the language so should be accepted by regular expression.
Examples of valid strings = {a, b, aaa, aba, aabba, aabbb,………}and all other strings that contain odd length strings.
inValid Strings:
These all strings are not part of the language, so should be rejected by our regular expression.
Examples of invalid strings = {aa, bb, ab, ba, aaaa, abba, abbb,……………..} and all other strings that does not contain odd length strings.
R.E = (a+b) (aa+bb+ab+ba)*
| Part 1 | Part 2 |
| (a+b) | (aa+bb+ab+ba)* |
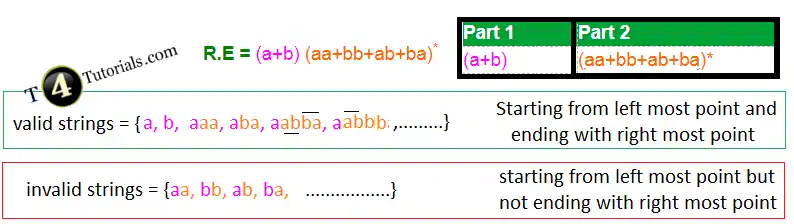
Part 1:
Restricting the machine to read only one odd alphabet so the machine can read only a or b.
Part 2:
Restricting the machine to read many times even length alphabet like a machine can read aa or bb or ab or ba and machine can read it many times by repeating the loop(*).
DFA for all odd-length strings
Write the Regular expression for the language of all even length strings but starts with a defined over ∑ = {a, b}?
Accepted Strings:
These all strings are the part of the language so should be accepted by regular expression.
Example strings = {aa, ab, aaab, aaab, aabb, abba, ababab………}and all other strings that are started with a and have even length.
Rejected Strings:
These all strings are not part of the language so should be rejected by regular expression.
Example strings = {a, b, bb, ba, bab, bab, bba, babb,……………..} and all other strings that do not contain odd length strings.
R.E = (ab + aa) (aa+bb+ab+ba)*
| Part 1 | Part 2 |
| (ab + aa) | (aa+bb+ab+ba)* |
Part 1:
Bounding the machine to read the only even alphabet and starting with a.
Part 2:
Bounding the machine to read many times even length alphabet like a machine can read aa or bb or ab or ba and machine can read it many times by repeating the loop(*).
Deterministic Finite Automata for the language of all even length strings but starts with a
Write the Regular expression for the language of all even length strings but ends with aa defined over ∑ = {a, b}?
Accepted Strings:
These all strings are part of the language so should be accepted by regular expression.
Example strings = {aa, baaa, bbaa, babaaa, …….}and all other strings that end with aa and have even length.
Rejected Strings:
These all strings are not part of the language so should be rejected by regular expression.
Example strings = {a, b, ab, ba, bb, abb, aba, bab, aab, bbab, abbb,……………..} and all other strings that do not contain even length strings and not end with aa.
R.E = (aa+bb+ab+ba)* aa
| Part 1 | Part 2 |
| (aa+bb+ab+ba)* | aa |
Part 1:
Bounding the machine to read many times even length alphabet like the machine can read aa or bb or ab or ba and machine can read it many times by repeating the loop(*).
Part 2:
Bounding the machine to read-only aa at the end.
List of 100+ Important Regular Expression
Regular Expression for Strings starts with a and ends with b and having an odd length string defined over {0,1}.
a( (a+b)((a+b)(a+b))* )b
valid strings={aab, abb,aaaab, aaabb, aabbb, abbbb, abaab,….}
Regular expression odd number of 1’s defined over {0,1}.
R.E = 0*(10*10*)*10*
Regular expression odd number of 0’s defined over {0,1}.
R.E = 1*(01*01*)*01*
Regular expression odd number of b’s defined over {a,b}.
R.E = a*(ba*ba*)*ba*
Regular expression odd number of a’s defined over {a,b}.
R.E = b*(ab*ab*)*ab*
Regular expression for every odd position is 1 defined over {0,1}
1(01)*
Regular expression for every odd position is 0 defined over {a,b}
0(10)*
Regular expression for every odd position is a defined over {a,b}
a(ba)*
Regular expression for every odd position is b defined over {a,b}
b(ab)*
More Examples of Regular Expression
- Regular Expression for no 0 or many triples of 0’s and many 1 in the strings.
- RegExp for strings of one or many 11 or no 11.
- A regular expression for ending with abb
- A regular expression for all strings having 010 or 101.
- Regular expression for Even Length Strings defined over {a,b}
- Regular Expression for strings having at least one double 0 or double 1.
- Regular Expression of starting with 0 and having multiple even 1’s or no 1.
- Regular Expression for an odd number of 0’s or an odd number of 1’s in the strings.
- Regular Expression for having strings of multiple double 1’s or null.
- Regular Expression (RE) for starting with 0 and ending with 1.
- RE for ending with b and having zero or multiple sets of aa and bb.
- A regular expression of the second last symbol is 1.
- RE for starting with 1 having zero or multiple even 1’s.
- Regular Expression for multiple a’s and multiple b’s.
- RE for exactly single 1 many 0’s |exactly single a many b.
- A regular expression for strings starting with aa and ending with ba.
- A regular expression for the language of all consecutive even length a’s.
- A regular expression for the language of all odd-length strings
- A regular expression for the language of all even length strings but ends with aa.
- A regular expression for the language of an odd number of 1s.
- A regular expression for the language of even length strings starting with a and ending with b in theory of automata.
- A regular expression for the language of all even length strings but starts with a.
- A Regular Expression for the Language of all strings with an even number of 0’s or even number of 1’s.
- A regular expression for the language of all those strings end with abb.
- A regular expression for string having must 010 or 101.
- Regular expression of strings begin with 110
Regular expression of strings begin and end with 110
Regular expression of strings containing exactly three consecutive 1’s. - A Regular Expression of all strings divisible by 4.
- A Regular Expression Strings that does not contain substring 110.
Tutorial: Regular Expression
A detailed tutorial of the regular expression is here in the link of regular expression tutorial. This page contains the practice questions of regular expressions with solutions.
Tutorial covering the topics
- Give a regular expression.
- Describe the strings of the regular expression.
- write a regular expression.
- create all strings from regular expression.
- Generate all strings from regular expression.
- Extract all strings from regular expression.
- Find all strings from regular expression.
- Examples of regular expression.
I welcome to all of you if you want to discuss about any topic. Researchers, teachers and students are allowed to use the content for non commercial offline purpose. Further, You must use the reference of the website, if you want to use the partial content for research purpose.
- What Is AssignCode and How Can It Help With Java Assignment? - May 27, 2021
- How to write a resume for a job search: Tips for applicants - May 11, 2021
- The Best Tool to Test the Speed of Your Internet - May 5, 2021